The Lawes of Motion
The Lawes of Motion
How solitary bodyes are moved.
Sect: 1[1]. There is an uniform extension, space, or expansion continued every way wthout bounds: in wch all bodyes are, each in severall ꝑts of space possessed {illeg} adequately felled by their ꝑts of it: wch ꝑts of space possesse {illeg} adequately filled by ym are their places. And their passing out of one place or ꝑt of space into another, through all ye intermediate space is their {p}|m|otion. Which motion is done wth more or lesse velocity acordingly {sic} as tis done through more or lesse space in equal times or through equall spaces in more or lesse time. But ye motion it selfe & ye force to pꝫsevere in yt motion is more or lesse accordingly as ye factus of ye bodys bulk into its velocity is more or lesse. And yt force is equivalent to that motion wch it is able to beget or destroy.
2[2]. The motion of a body tends one way 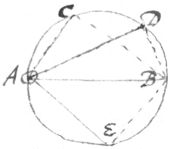 directly & severall other ways obliqly. As if ye body A move directly towards ye point B it also moves obliquely towards all ye lines BC, BD, BE & wch passe through yt point B: & shall arrive {illeg}to yt|ym| all i|a|t ye same time. Whence its velocity towards ym is in such proportion as its distance from them yt is, as AB, C|A|C, AD, AE &c.
directly & severall other ways obliqly. As if ye body A move directly towards ye point B it also moves obliquely towards all ye lines BC, BD, BE & wch passe through yt point B: & shall arrive {illeg}to yt|ym| all i|a|t ye same time. Whence its velocity towards ym is in such proportion as its distance from them yt is, as AB, C|A|C, AD, AE &c.
3[3]. If a body A move towards B wth  the velocity R, & by ye way hath some new force done to it wch had ye body rested would have propeld|{e}|d it towards C wth ye velocity S. Then making AB∶AC∷R∶S, & Completing ye Parallelogram BC ye body shall move in ye Diagonall AD & arive {sic} at ye point D in ye same time wth this compound motion in ye same time it would have arrived at ye point B wth its single motion.
the velocity R, & by ye way hath some new force done to it wch had ye body rested would have propeld|{e}|d it towards C wth ye velocity S. Then making AB∶AC∷R∶S, & Completing ye Parallelogram BC ye body shall move in ye Diagonall AD & arive {sic} at ye point D in ye same time wth this compound motion in ye same time it would have arrived at ye point B wth its single motion.
4[4] In every body there is a certaine point, called its center of motion about wch if ye body bee any way circulated ye endeavours of its ꝑts every way from ye center are exactly counterpoised by opposite endeavours. And ye progressive motion of ye body is ye same wth ye motion of this its center wch always moves in a streight line & uniformly wn ye body is free from occursions wth other bodys{illeg}|.| And so doth ye common center of two bodys; wch is found by dividing ye distance twixt their propper centers in reciprocall proportion to their bulk. And so ye common center of 3 or more bodys &c. And all ye lines passing through these centers of motion are axes of motion.
5[5]. The angular quantity of a bodys circular 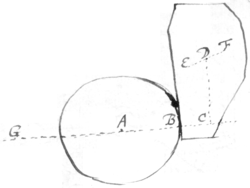 motion & velocity is more or lesse accordingly as ye body makes one revolution in more {or} lesse time but ye reall quantity of its circular motion is more or lesse accordingly as ye body hath more or lesse power & force to pꝫsevere in yt motion; wch motion divided by ye bodys bulke is more the reall quantity of its circular velocity. Now to know ye reall quantity of a bodys circular {illeg}|m|otion & velocity about any given axis EF; Suppose it hung upon ye two end E & F of yt axis as upon two poles: And yt another globular body of ye same bignesse, whose center is A, is so placed yt ye circulating body shall hit it in ye {illeg}|p|oint F & strike it away in ye line BAG (wch lyeth in ye same plane wth one of ye circles described about ye axis EF) & thereby just loose all its owne motion. Then hath ye {illeg} Globe gotten ye same quantity of progressive motion & velocity wch ye other had of circular, {wch} /its\ velocity being ye same wth yt of ye point C wch describes a circle touching ye line BG. The Radius DC of wch circle I may therefore call ye radius of Circular motion or velocity about yt axis EF. And ye circle described wth ye said Radius of Circulation in that plane wch cuts ye axis EF perpendicularly in ye center of motion I call ye Equator of circulation about that axis, and those circles wch passe through ye poles, me{d}ridians &c.
motion & velocity is more or lesse accordingly as ye body makes one revolution in more {or} lesse time but ye reall quantity of its circular motion is more or lesse accordingly as ye body hath more or lesse power & force to pꝫsevere in yt motion; wch motion divided by ye bodys bulke is more the reall quantity of its circular velocity. Now to know ye reall quantity of a bodys circular {illeg}|m|otion & velocity about any given axis EF; Suppose it hung upon ye two end E & F of yt axis as upon two poles: And yt another globular body of ye same bignesse, whose center is A, is so placed yt ye circulating body shall hit it in ye {illeg}|p|oint F & strike it away in ye line BAG (wch lyeth in ye same plane wth one of ye circles described about ye axis EF) & thereby just loose all its owne motion. Then hath ye {illeg} Globe gotten ye same quantity of progressive motion & velocity wch ye other had of circular, {wch} /its\ velocity being ye same wth yt of ye point C wch describes a circle touching ye line BG. The Radius DC of wch circle I may therefore call ye radius of Circular motion or velocity about yt axis EF. And ye circle described wth ye said Radius of Circulation in that plane wch cuts ye axis EF perpendicularly in ye center of motion I call ye Equator of circulation about that axis, and those circles wch passe through ye poles, me{d}ridians &c.
6[6]. A body circulates about one axis (as PC) 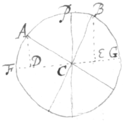 directly & about severall other axes (as AC, BC, &c) obliquely. And ye angular quantity of its circulations about those axes (PC, AC, BC &c) are as ye sines (PC, AD, BE, &c) of ye angles wch those axes make wth ye Equator (FG) of ye principall & direct axis (PC).
directly & about severall other axes (as AC, BC, &c) obliquely. And ye angular quantity of its circulations about those axes (PC, AC, BC &c) are as ye sines (PC, AD, BE, &c) of ye angles wch those axes make wth ye Equator (FG) of ye principall & direct axis (PC).
7[7]. If a body circulates about ye axis AC wth  ye angular quantity of velocity R: & some new force is done to it, wch, if ye body had rested, would have made it circulate about another axis BC, wth ye angular quantity of velocity S. Then in ye plane of ye two axes, & in one of those two opposite angles (made by ye axes) in wch ye two circulations are contrary one to another, (as in ye angle ACB). I find such a point P from wch ye perpendic{illeg}{la}rs \(PK, PH)/ let fall to those axes are bee reciprocally proportional to ye angular velocitys about those axes, (yt is PK∶PH∷R∶S). And drawing ye line PC, it shall bee ye new {ab} axis about wch ye compound motion is pꝫformed. And ye summe of & {CD} when ye perpendiculars PH & PK fall on divers sides of ye axis PC, otherwise their difference, is ye angular quantity of circulation about yt axis: Wch in ye angle tends contrary to ye circulation about ye axis
ye angular quantity of velocity R: & some new force is done to it, wch, if ye body had rested, would have made it circulate about another axis BC, wth ye angular quantity of velocity S. Then in ye plane of ye two axes, & in one of those two opposite angles (made by ye axes) in wch ye two circulations are contrary one to another, (as in ye angle ACB). I find such a point P from wch ye perpendic{illeg}{la}rs \(PK, PH)/ let fall to those axes are bee reciprocally proportional to ye angular velocitys about those axes, (yt is PK∶PH∷R∶S). And drawing ye line PC, it shall bee ye new {ab} axis about wch ye compound motion is pꝫformed. And ye summe of & {CD} when ye perpendiculars PH & PK fall on divers sides of ye axis PC, otherwise their difference, is ye angular quantity of circulation about yt axis: Wch in ye angle tends contrary to ye circulation about ye axis
8[8]. Every body keepes ye same reall quantity of circular motion & velocity so long as tis not opposed by other bodys. And it keeps ye same axis too if ye endeavour from ye axis wch ye two opposite quarters twixt ye Equator & every meridian of motion have, bee exactly counterpoised by {on}|th|e opposite endeavours of ye 2 side quarters; & yn also its axis doth always keepe parallel to it selfe. But if ye said endeavours from ye axis bee not exactly counterpoised by such opposite endeavours: yn for want of such counterpoise ye pꝫvalent ꝑts shall by little & little get further from ye axis & draw nearer & nearer to such a Counterpoise, but shall {illeg}|n|ever bee exactly counterpoised. And as ye axis is continually moved in ye body, so it continually moves in ye space too wth some kind or other of spirall motion; always drawing nearer & nearer to a center or parallelisme wth it selfe, but never attaining to it. Nay tis so far from ever keeping parallel to it sel selfe, yt it shall never bee twice in ye same position.
How Bodys are Reflected.
9[9]. Suppose ye bodys A & α did move in 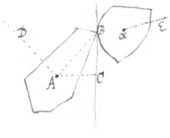 ye lines DA & Eα till they met in the point B: yt BC is ye plane wch toucheth them in ye point of contact B: yt ye velocity of ye Body A towards ye said plane of contact is B, & ye motion AB; & yt ye change wch is made \by reflection/ in yt velocity & motion is X & AX. Suppose also yt from ye body A its center of motion two lines are drawne ye one AB to ye point of contact ye other AC to ye plane of Contact: yt ye intercepted line BC is F: that ye axis of motion wch is perpendicular to ye plane ABC & its Equator are called ye axis & Equator of reflected circulation: yt ye radius of yt Equator is G: yt ye reall quantity of velocity about yt axis is D, & ye AD: yt ye change wch reflection {m}akes in yt velocity & motion is y & AY: And yt ye correspondent lines & motions of ye other body α are β, αβ, ξ, αξ, φ, γ, δ, αδ, ν & αν. Lastly for brevity sake suppose yt . And . Observing yt at ye time of reflection if in either body ye center of motion doth move from ye plane of contact, or those ꝑts of it nearest ye point of contact doe circulate from ye plane of contact: yn ye said motion is to bee esteemed negative & ye signe of its velocity B, β, D or δ must bee made negative in ye valor of Q.
ye lines DA & Eα till they met in the point B: yt BC is ye plane wch toucheth them in ye point of contact B: yt ye velocity of ye Body A towards ye said plane of contact is B, & ye motion AB; & yt ye change wch is made \by reflection/ in yt velocity & motion is X & AX. Suppose also yt from ye body A its center of motion two lines are drawne ye one AB to ye point of contact ye other AC to ye plane of Contact: yt ye intercepted line BC is F: that ye axis of motion wch is perpendicular to ye plane ABC & its Equator are called ye axis & Equator of reflected circulation: yt ye radius of yt Equator is G: yt ye reall quantity of velocity about yt axis is D, & ye AD: yt ye change wch reflection {m}akes in yt velocity & motion is y & AY: And yt ye correspondent lines & motions of ye other body α are β, αβ, ξ, αξ, φ, γ, δ, αδ, ν & αν. Lastly for brevity sake suppose yt . And . Observing yt at ye time of reflection if in either body ye center of motion doth move from ye plane of contact, or those ꝑts of it nearest ye point of contact doe circulate from ye plane of contact: yn ye said motion is to bee esteemed negative & ye signe of its velocity B, β, D or δ must bee made negative in ye valor of Q.
10[10]. The velocitys B, β, D & δ & they only are directly opposed & changed in Reflection; & yt according to these rules {illeg} . . . & . Which mutations X ξ Y & ν tend all of them from ye plane of Contact. And these four rules I gather thus: The whole velocity of ye two points of contact towards one another perpendicularly to ye plane of contact is Q (arising ꝑtly from ye bodys progressio|v|e velocity B & β & ꝑtly from their circular D & δ): And ye same points are reflected one from another wth ye same quantity of such velocity. So yt ye whole change of all yt their velocity wch is perpendicular to ye plane of contact is Q. Which change must bee distributed amongst ye foure opposed velocitys B, β D & δ proportionably to ye easinesse (or smallnesse of resistance) wth wch those velocitys are changed, yt is, proportionably to , , , & . Soe yt . that is . . . & .
11[11]. Now if any two reflecting bodys A & α, wth ye quantity of their progressive & angular motions; & their position at their meeting & consequently their point & plane of contact &: be given: to know how those bodys shall bee reflected, First {illeg} find B & β by sec 2. Then ye lines F & φ by sec & ye axis of reflected circulation by sec 9. yn & their Radij G & γ by sec 5. Then their angular quantity of velocity about ye axes of reflected circulation by sec 6, & ye reall quantity D & δ by sec 5. Then P & Q by sec 9 Then X, ξ, Y & ν by sec 10. Then ye bodys new progressive determinations & velocitys by sec 3. Then ye angular quantity of yt circulation \(Y & ν)/ wch is generated by reflection by sec 5. And lastly ye new axes & angular quantity of velocity about ym by sec 7.
Some Observations about Motion.
Only those bodyes which are absolutely hard are exactly reflected acording to those rules. Now the bodyes here amongst us (being an aggregate of smaller other bodyes) haue a relenting softnesse & springynesse, wch makes their contact be for some time & in more points then one. And ye touching surfaces during ye time of contact doe slide one upon another more or lesse or not at all acording to their roughnesse. And few or none of these bodyes haue a springynesse soe strong, as to force them one from another wth ye same vigor that they came together. Besides yt their motions are continually impeded & slackened by ye mediums in wch they move. Now hee yt would prescribe rules for ye reflections of these compound bodies, must consider in how many points ye two bodies touch at their meeting, ye position & pression of every point, wch their planes of contact &c: & how all these are varyed every moment during ye time of contact by ye more or lesse relenting softnesse or springynesse of those bodies & their various slidings. And also what effect ye air or other mediums compressed betwixt ye bodies may haue.
2 These are some cases of Reflections of bodies absolutely hard to which these rules extend not: As when two bodies meet wth their angular point, or in more points then one at once; Or with their superficies. But these cases are rare.
3 In all reflections of any bodies wth|wt| ever this rule is true that ye comon center of t{i}|w|o or more bodies changeth not its state of motion or rest by ye reflection of those bodies one amongst another.
4 Motion may be lost by reflection. As 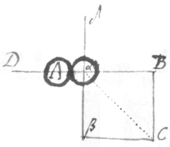 if two equall {bodies} Globes A & α wth equall motions from D & δ done in the perpendicular lines DA & δα, hit one another when the center of ye body α is |in| ye line DA. Then ye body A shall loose all its motion & yet ye motion of α is not doubled. For completing ye square Bβ, ye body α shall move in ye Diagonall αC, & arrive at C but at ye same time it would haue arrived at β wthout reflection. see ye third section.
if two equall {bodies} Globes A & α wth equall motions from D & δ done in the perpendicular lines DA & δα, hit one another when the center of ye body α is |in| ye line DA. Then ye body A shall loose all its motion & yet ye motion of α is not doubled. For completing ye square Bβ, ye body α shall move in ye Diagonall αC, & arrive at C but at ye same time it would haue arrived at β wthout reflection. see ye third section.
5 Motion may be gained by reflection. for if ye body For if the body α return wth ye same motion back again from C to α. The two bodyes A & α after reflection shall regain ye same equall motions in ye lines AD & αδ (though backwards) wch they had at first.
[1] Of Place motion velocity & force.
[2] wth wt velocity a body moves severall ways at once.
[3] How two progressive motions are joyned into one.
[4] Of centers & axes of motion & ye motion of those centers.
[5] Of circular motion and velocity about those axes.
[6] Wth wt velocity a body circulates about severall axes at once.
[7] How two circular motions are joyned into one.
[8] In wt cases a circulating body pꝫsevere in ye same state & in wt it doth not.
[9] Some names & letters defined.
[10] The Rule for Reflection
[11] The conclusion. In wt method ye precedent rules must be used.
